Magnitude and Phase Spectra, Ideal Filtering

Effect of Ideal Filtering:

The Inverse Fourier transform of Y(w) = X(w) for |w| < W, and Y(w) = 0 for |w| > W is

y(t) = (1/2p) _{_-W/}|^{^/W}X(w)e^jwtdt

Therefore the time signal corresponding to the ideal filtered spectra

|Y(w)| = |X(w)| for |w| < W, |Y(w)| = 0 for |w| >W

<Y(w) = <X(w) for |w| < W, <Y(w) = 0 for |w| > W

is related to x(t) in a rather complicated way.

Compare the magnitude spectrum of X(w) with the filtered magnitude spectrum of Y(w)

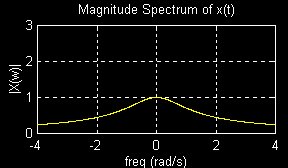
and the phase spectrum of X(w) with the filtered phase spectrum of Y(w)

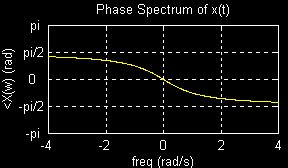
to the corresponding time signals

x(t) = e^-tu(t)
y(t) = (1/2p) _{_-W/}|^{^/W}X(w)e^jwtdt